The Monte Carlo method is a strategy designed to improve the chances of winning in online casino games.
It is grounded in probability theory and focuses specifically on managing and increasing the amount of bets.
This page outlines the detailed steps, simulations, benefits, and drawbacks of using the Monte Carlo method.
What you’ll find in this guide:
- How the Monte Carlo method applies to games offering 3x or 2x payouts.
- Techniques for managing your bets using notes and mobile apps.
- How to aim for substantial profits without letting your betting amounts escalate uncontrollably.
What is the Monte Carlo Method of Play?
The Monte Carlo method is a betting strategy applicable to crash games as well as traditional casino table games.
At its core, it is a money management system where bets are adjusted—either increased or decreased—based on specific rules. A series of bets is completed with the ultimate aim of generating a profit.
While this approach may seem daunting to beginners due to the need for detailed record-keeping, the process itself is not overly complex. With some familiarity and practice, it can be implemented effectively and with confidence.
Games Where the Monte Carlo Method Can Be Applied
The Monte Carlo method is suitable for any casino games offering payouts of 2x or 3x. Here’s a closer look at how it applies to these types of games.
Casino Games with 3x Payouts (1/3 Win Rate)
The Monte Carlo method is particularly effective in games where a correct bet yields triple the payout.
This makes it most commonly used in the ‘dozen bet’ and ‘column bet’ options in roulette.
- Dozen Bets: These involve placing your wager on one of the three groups of 12 numbers (1–12, 13–24, or 25–36).
- Column Bets: Here, you bet on one of the three horizontal groups of 12 numbers (e.g., 1, 4, 7, etc.).
Additionally, there’s flexibility to bet on any 12-number segment, such as numbers 1–6 or 31–36.
For all these bets, the win rate is roughly one in three.
Games with 2x Payouts (1/2 Win Rate)
The Monte Carlo method can also be employed in games offering double payouts for a winning bet.
This includes popular table games such as:
- Crash
- Roulette (Red/Black)
- Baccarat (Player Bet)
- Sic Bo (Big/Small)
- Dragon Tiger
If you’re unfamiliar with dozen or column bets in roulette, these simpler 2x payout games are a great starting point.
However, there is a notable downside: even if you successfully complete a betting sequence, there’s a chance you won’t make a profit.
More details on this drawback are provided in the Disadvantages section.
How to Bet Using the Monte Carlo Method
Here is a step-by-step guide to betting with the Monte Carlo method.
As this system is somewhat complex, beginners are strongly advised to practise in free demo modes first, gaining confidence in the technique before wagering real money in live casino games.
To begin with, it can be useful to practise on non-live roulette games, as these do not impose time limits on placing bets.
In the next section, you will see a simulation of the Monte Carlo method in action, illustrating how your bets progress and what kind of profit or loss you might anticipate.
Procedure of the Monte Carlo Method
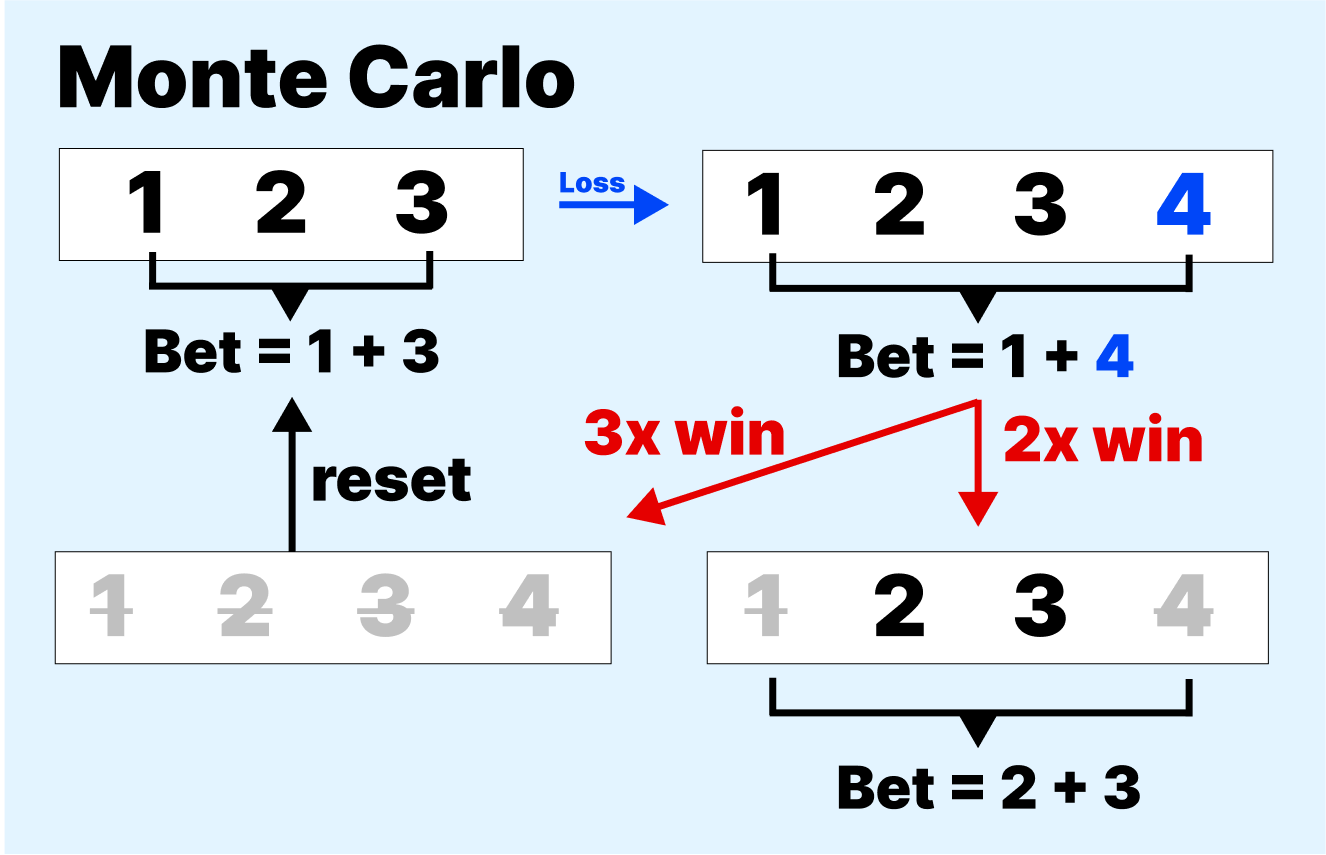
The Monte Carlo method employs a ‘sequence of numbers’ to determine the betting amount.
Unless you are exceptionally skilled at mental arithmetic, make sure to have your notes prepared.
Use a pen and paper or a note-taking app to keep track.
Furthermore, owing to the slightly intricate rules, take your time going through the procedure until you are confident with it, so as to avoid making mistakes.
1. Determine the Amount of One Unit
First, decide on the amount of ‘one unit’, which represents the betting amount.
However, note that the Monte Carlo method begins with a starting wager of ‘4 units’. Therefore, if you choose a higher value for one unit, as with other strategies, you are likely to end up placing a high bet.
In this explanation, we will assume that ‘1 unit = 1 dollar’.
2. State the First Number Sequence ‘1, 2, 3’
Write the number sequence as ‘1, 2, 3’. Leave the right-hand side of the note blank, as additional numbers will be added there as the sequence progresses.
The leftmost number in this sequence represents one unit, and if one unit equals $10, you would write ‘10, 20, 30’.
3. Start with the Numbers Added at Both Ends
Place the next bet, with the amount always being the ‘sum of the leftmost and rightmost numbers’ in the sequence.
Thus, the first bet is calculated as $1 + $3 = $4.
If you win on the first bet, the Monte Carlo method is deemed successful, regardless of whether you receive a 3x or 2x payout.
In this case, the sequence is reset, and the next set begins with the original sequence ‘1, 2, 3’.
4. If You Lose, Add the Bet Amount to the Right and Bet Again, Adding the Numbers from Both Ends
If you lose, the amount of the bet is written on the right-hand side of the number line.
Example: If you place a $4 bet on the number line ‘1, 2, 3’ and lose, the sequence becomes → ‘1, 2, 3, 4’.
You then place another bet. As before, the bet amount is the sum of the numbers at the left and right ends of the sequence. In the example above, 1 + 4 = $5.
Continue this process, always adding the amount of the current bet to the right end of the number line whenever you lose.
5. If You Win, Remove the Numbers from Both Ends of the Number Line
If you win, the steps to follow differ depending on whether the game offers 3x payouts or 2x payouts.
- If You Win in a 3x Payout Game
The process is to ‘remove the two numbers at both ends’ of the number sequence.
Example: If you win with the number sequence ‘1, 2, 3, 4, 5, 6’, it becomes → ‘3, 4’.
Note that if the sequence contains three or fewer numbers, all numbers are removed.
- If You Win with a 2x Payout
The process is to ‘remove one number at each end’ of the number sequence.
Example: If you win with the number sequence ‘1, 2, 3, 4, 5, 6’, it becomes → ‘2, 3, 4, 5’.
Continue this process, always removing numbers from the sequence after a win.
6. Profit Is Confirmed When There Are No More Numbers to Erase or Only One Number Left to Erase
Each round, if you win, you eliminate a number in the sequence, and if you lose, you add to the sequence.
The Monte Carlo method is successful when all or only one number is erased.
- In the case of a 3x payout, the money is always positive upon success.
- In the case of a 2x payout, the profit is confirmed when all the numbers are gone, but not when there is only one left.
If the Monte Carlo method is successful, the entire sequence of numbers is reset, starting from the first ‘1, 2, 3’.
Explanation of How to Describe the Monte Carlo Sequence of Numbers Based on Practice
The rules of the Monte Carlo method have been explained so far, but many people might still struggle to visualise how it is actually used. For this reason, a simulation of the Monte Carlo method in practice is provided.
In this case, the simulation involves placing a bet on roulette, specifically on the 1–12 dozen bet, with 1 unit equal to 1 USD. In the first five games, five consecutive losses occur, followed by two consecutive wins immediately afterwards.
The table below outlines the history of the number sequence, along with the wagers and profit or loss, allowing you to understand how the Monte Carlo method works in practice. Before reviewing the table, it is recommended that you create your own number line to ensure you are following the sequence correctly.
| # | Wager | Win/Loss | Gain/Loss |
|---|---|---|---|
| 1・2・3 | $4 | Loss | -$4 |
| 1・2・3・4 | $5 | Loss | -$9 |
| 1・2・3・4・5 | $6 | Loss | -$15 |
| 1・2・3・4・5・6 | $7 | Loss | -$22 |
| 1・2・3・4・5・6・7 | $8 | Loss | -$30 |
| 1・2・3・4・5・6・7・8 | $9 | Win | -$12 |
| $9 | Win | +$6 | |
| – | – | – |
The sequence begins with ‘1, 2, 3’, and after losing five consecutive games, the sequence extends to eight numbers. When the first win is achieved, the numbers 1, 2, 7, and 8 are removed, leaving only 3, 4, 5, and 6.
After the next win, all remaining numbers are cleared, resulting in a profit of 6 USD.
This simulation demonstrates that the Monte Carlo method has the advantage of keeping betting amounts from rising excessively during a losing streak while still yielding a substantial profit. Even with 1 unit equal to just 1 USD, the result is comparable to other betting strategies.
However, although some people may find it easy to mentally keep track of the sequence in a short game, taking notes is essential. In longer games, it becomes difficult to recall which numbers have been added and which have been erased. Without proper record-keeping, mistakes in your betting calculations are likely.
Monte Carlo Method: Record of Successes
We hope that the explanation so far has helped you understand that a sequence of numbers is successful (clear) if it disappears or only one remains.
Now we will explain how to put this into practice in earnest.
- First, decide on the number of times you want to clear the record and continue until you achieve it. In this case, the goal is to clear five times and aim to achieve it.
- The upper limit of the bankroll is set at $100, and if it runs out or is insufficient, the game is over.
If the amount of money you have invested becomes too high and it becomes difficult to clear the game, you must cut your losses to determine the amount of money you have lost and start again from the beginning. At that time, the maximum amount of money you can play with will be set again.
We have used a dozen bets (3x) on Red Tiger’s Roulette. The table below records the practice.
| # | Sequence | Betting Amount | Win/Loss | Profit | Balance |
|---|---|---|---|---|---|
| 1 | 1・2・3 | 4 | ✕ | -4 | 96 |
| 2 | 1・2・3・4 | 5 | ✕ | -5 | 91 |
| 3 | 6 | ◯ | 18 (+12) | 103 | |
| 4 | 1・2・3 | 4 | ✕ | -4 | 99 |
| 5 | 1・2・3・4 | 5 | ✕ | -5 | 94 |
| 6 | 1・2・3・4・5 | 6 | ✕ | -6 | 88 |
| 7 | 1・2・3・4・5・6 | 7 | ✕ | -7 | 81 |
| 8 | 8 | ◯ | 24 (+16) | 97 | |
| 9 | 8 | ◯ | 24 (+16) | 113 | |
| 10 | 4 | ◯ | 12 (+8) | 121 | |
| 11 | 4 | ◯ | 12 (+8) | 129 | |
| 12 | 1・2・3 | 4 | ✕ | -4 | 125 |
| 13 | 1・2・3・4 | 5 | ✕ | -5 | 120 |
| 14 | 1・2・3・4・5 | 6 | ✕ | -6 | 114 |
| 15 | 7 | ◯ | 21 (+14) | 128 | |
| 16 | 3・4 | 7 | ✕ | -7 | 121 |
| 17 | 3・4・7 | 10 | ✕ | -10 | 111 |
| 18 | 13 | ◯ | 39 (+26) | 137 |
In 18 plays, I have successfully completed the game five times. The time played is about 20 minutes.
The fund has increased from $100 to $137.
Examining the Differences Between the 3x and 2x Payouts of the Monte Carlo Method
Next, the Monte Carlo method is simulated using both the 2x and 3x payouts of roulette. The results and impressions of applying the Monte Carlo method are explained below.
Rules for This Monte Carlo Method Verification
Let me explain the rules for the Monte Carlo verification:
- The ‘French Roulette’ free play at LTC Casino was used. The verification was carried out twice for 20 games each. The verification ended after 20 games with any result.
- The first simulation was conducted using a ‘triple payout’, while the second simulated a ‘double payout’.
- The first simulation involved the ‘1–12’ (dozen bet), and the second involved the ‘red’ bet. The bet amount was adjusted according to the Monte Carlo rules.
- The starting amount was USD 500.
| Games played | French Roulette |
| Number of games | 20 |
| Starting amount | $500 |
Monte Carlo Method: Verification of the 3x Payout
In the 3x payout simulation, the trend was positive initially but managed to handle losing streaks effectively.
Since the Monte Carlo method ensures ‘always positive on every third win’ and ‘always positive if you win at least once in five games (as you can eliminate four numbers on a win)’, the results averaged out, yielding a reasonable profit despite losses. The method performed well, demonstrating its effectiveness.
However, there was a five-game losing streak from the 15th game, followed by a win in the 20th game. Unfortunately, the set had to end with numbers remaining. The overall result was 7 wins, 13 losses, +$21.
| # | Pre-sequences | Wager | Win/Loss | Post-sequences | Profit |
|---|---|---|---|---|---|
| 1 | 1 2 3 | $4 | WIn | 2 | $8 |
| 2 | 1 2 3 | $4 | WIn | 2 | $16 |
| 3 | 1 2 3 | $4 | Loss | 1 2 3 4 | $12 |
| 4 | 1 2 3 4 | $5 | WIn | – | $22 |
| 5 | 1 2 3 | $4 | Loss | 1 2 3 4 | $18 |
| 6 | 1 2 3 4 | $5 | Loss | 1 2 3 4 5 | $13 |
| 7 | 1 2 3 4 5 | $6 | WIn | 3 | $25 |
| 8 | 1 2 3 | $4 | Loss | 1 2 3 4 | $21 |
| 9 | 1 2 3 4 | $5 | Loss | 1 2 3 4 5 | $16 |
| 10 | 1 2 3 4 5 | $6 | Loss | 1 2 3 4 5 6 | $10 |
| 11 | 1 2 3 4 5 6 | $7 | WIn | 3 4 | $24 |
| 12 | 3 4 | $7 | Loss | 3 4 7 | $17 |
| 13 | 3 4 7 | $10 | Loss | 3 4 7 10 | $7 |
| 14 | 3 4 7 10 | $13 | WIn | – | $33 |
| 15 | 1 2 3 | $4 | Loss | 1 2 3 4 | $29 |
| 16 | 1 2 3 4 | $5 | Loss | 1 2 3 4 5 | $24 |
| 17 | 1 2 3 4 5 | $6 | Loss | 1 2 3 4 5 6 | $18 |
| 18 | 1 2 3 4 5 6 | $7 | Loss | 1 2 3 4 5 6 7 | $11 |
| 19 | 1 2 3 4 5 6 7 | $8 | Loss | 1 2 3 4 5 6 7 8 | $3 |
| 20 | 1 2 3 4 5 6 7 8 | $9 | WIn | 3 4 5 6 | $21 |
Highlights
- Game 7: Breaking a Two-Game Losing Streak
This game demonstrates the method’s high success rate, which ensures profits even after the third win. While the initial success rate is high, consecutive losses demand at least one win in three games. - Games 8–14: Achieving Profit with 2 Wins and 5 Losses
Despite losses, a profit of +$8 was achieved, showcasing the resilience of the Monte Carlo method. - Games 15–20: Struggling Through Five Consecutive Losses
Losing streaks proved challenging, and the game ended with more than two numbers remaining in the sequence, making the set incomplete.
Monte Carlo Method: Verification of the 2x Payout
This simulation focused on a 2x payout. Although consecutive losses are less likely than with 3x payouts, the method faced six consecutive losses starting from game three. The number sequence expanded and contracted repeatedly, and the sequence was successfully eliminated after four consecutive wins in games 12–15. However, this resulted in $0 profit for that set.
Nevertheless, the simulation concluded with 10 wins, 10 losses, and +$14. This demonstrated the Monte Carlo method’s resilience, tolerating extended losing streaks, where flat betting would not have been profitable.
| # | Pre-sequences | Wager | Win/Loss | Post-sequences | Profit |
|---|---|---|---|---|---|
| 1 | 1 2 3 | $4 | Win | 2 | $4 |
| 2 | 1 2 3 | $4 | Win | 2 | $8 |
| 3 | 1 2 3 | $4 | Loss | 1 2 3 4 | $4 |
| 4 | 1 2 3 4 | $5 | Loss | 1 2 3 4 5 | -$1 |
| 5 | 1 2 3 4 5 | $6 | Loss | 1 2 3 4 5 6 | -$7 |
| 6 | 1 2 3 4 5 6 | $7 | Loss | 1 2 3 4 5 6 7 | -$14 |
| 7 | 1 2 3 4 5 6 7 | $8 | Loss | 1 2 3 4 5 6 7 8 | -$22 |
| 8 | 1 2 3 4 5 6 7 8 | $9 | Loss | 1 2 3 4 5 6 7 8 9 | -$31 |
| 9 | 1 2 3 4 5 6 7 8 9 | $10 | Win | 2 3 4 5 6 7 8 | -$21 |
| 10 | 2 3 4 5 6 7 8 | $10 | Loss | 2 3 4 5 6 7 8 10 | -$31 |
| 11 | 2 3 4 5 6 7 8 10 | $12 | Loss | 2 3 4 5 6 7 8 10 12 | -$43 |
| 12 | 2 3 4 5 6 7 8 10 12 | $14 | Win | 3 4 5 6 7 8 10 | -$29 |
| 13 | 3 4 5 6 7 8 10 | $13 | Win | 4 5 6 7 8 | -$16 |
| 14 | 4 5 6 7 8 | $12 | Win | 5 6 7 | -$4 |
| 15 | 5 6 7 | $12 | Win | 6 | $8 |
| 16 | 1 2 3 | $4 | Win | 2 | $12 |
| 17 | 1 2 3 | $4 | Loss | 1 2 3 4 | $8 |
| 18 | 1 2 3 4 | $5 | Win | 2 3 | $13 |
| 19 | 2 3 | $5 | Win | – | $18 |
| 20 | 1 2 3 | $4 | Loss | 1 2 3 4 | $14 |
Highlights
- Game 8: Six Consecutive Losses
Losing streaks, though less probable for 2x payouts, can lead to sharp sequence increases. Here, the sequence grew to nine numbers, increasing risk. - Games 12–15: Four Consecutive Wins Yielded No Profit
The sequence was eliminated, but the set concluded with no profit, highlighting the challenge of achieving profitability with a double payout. - Games 16–20: Resilience with Final Gains
A consistent win rate helped recover from earlier losses, securing a modest profit of +$14.
Verification Results of the Monte Carlo Method
The Monte Carlo method demonstrated a reasonable profit for both 3x and 2x payouts, maintaining stability even during extended losing streaks.
- Payout Comparison
The 3x payout ensured more significant profits due to higher returns per win, while the 2x payout demonstrated steadier results despite smaller margins. - Psychological Advantage
The slower increase in wager amounts during losing streaks provided a sense of security, as ‘a profit is made if you win one in five games (3x payout)’. However, longer sequences in losing streaks proved challenging.
| Payout | Win/Loss | Profit |
|---|---|---|
| 3x | 7 wins, 13 losses | +$21 |
| 2x | 10 wins, 10 losses | +$14 |
Script for Crash Game
Here is a script for the Crash game that automates the Monte Carlo system.
var config={betAmount:{value:1,type:"number",label:"Bet Amount"},payout:{value:2,type:"radio",label:"Payout Multiplier",options:[{value:2,label:"2x Payout"},{value:3,label:"3x Payout"}]}};function main(){const e=[1,2,3];let o=[...e];const t=config.betAmount.value,n=config.payout.value;let l=0;log.info("Starting Monte Carlo System"),log.info("Initial sequence: "+o.join(",")),game.on("GAME_STARTING",(()=>{0!==o.length&&1!==o.length||(log.info("Sequence completed. Resetting to initial sequence."),o=[...e],log.info("Reset sequence: "+o.join(",")));const i=t*(o[0]+o[o.length-1]);log.info(`Placing bet: ${i} with payout ${n}x`),game.bet(i,n).then((e=>{if(e>=n){const t=i*(e-1);l+=t,log.success(`Win! Profit: ${t}. Cumulative Profit: ${l}`),2===n?(o.shift(),o.pop()):3===n&&(o.shift(),o.shift(),o.pop(),o.pop()),log.info("Updated sequence: "+o.join(","))}else l-=i,log.error(`Loss! Cumulative Profit: ${l}`),o.push(o[0]+o[o.length-1]),log.info("Updated sequence: "+o.join(","))})).catch((e=>{log.error("Bet failed: "+e),game.stop()}))}))}Learn how to add and run the script on BC.Game:
- Sign up at BC.Game.
- Go to ‘Auto’ Betting Mode: In the BC Originals Crash game, switch to “Auto” to see different crash game scripts.
- Add a Script: Press the Add Script button, put in the code for the script you want to start the automated betting, give it a name and press the Save button.
- Run Script: Turn on the script to automatically bet and possibly increase your cryptocurrency.
Advantages of the Monte Carlo Method
Although the Monte Carlo method is slightly more difficult to manage, once mastered, it creates the impression that you are ‘beating the online casino’ and enables you to play table games with greater confidence.
The Monte Carlo method is a betting system with many enthusiasts, as its advantages and disadvantages are well balanced. The following section describes the advantages of using the Monte Carlo method, including how it differs from other strategies.
You can play a steady game with a low spike in betting value
Unlike the Martingale method, which doubles your bet when you lose, or the Cocomo method, which adds the previous two bets together, the Monte Carlo method does not significantly increase your bet, even if you experience a losing streak.
As an extreme example, if you start with $1 per unit and immediately lose 20 consecutive bets, you only need to bet $204. By comparison, the Cocomo method requires about $10,000, and the Martingale method demands more than $2,000,000.
The advantage of the Monte Carlo method is that the betting amount does not escalate rapidly, enabling you to earn steady profits with lower psychological pressure. This is a major benefit for a strategy that focuses on defensive skills.
| # of consecutive losses | Martingale | Cocomo | Monte Carlo |
|---|---|---|---|
| 1 | $1 | $1 | $4 |
| 5 | $16 | $5 | $8 |
| 10 | $512 | $55 | $13 |
| 15 | $3072 | $377 | $18 |
Can be used even with a small amount of funds
Unlike the Martingale and Cocomo methods, where a large amount of funds is directly linked to a high level of defence, the Monte Carlo method can be used with minimal funds.
As a strategy that aims to achieve overall profits by repeatedly winning and losing, with only limited increases in bets when losing, the Monte Carlo method is less likely to exhaust your funds. Consequently, it can adapt to some extent, even when encountering a sudden series of losses.
Since defensive money systems inevitably require increased bets when losing, the Monte Carlo method’s ability to function effectively with low funds is a highly significant advantage.
Profit on success is high
Strategies focusing primarily on defensive skills tend to generate lower profits when winning. However, with the Monte Carlo method, even if you set 1 unit as your initial wager, it represents ‘4 units’.
For instance, even if 1 unit = $1, a first-time win yields a profit of $4 for a 2x payout and $8 for a 3x payout. Despite being a defensive strategy, which is generally not optimised for consecutive wins, the Monte Carlo method allows for substantial profit, making it practical.
The Monte Carlo Method is highly effective
The Monte Carlo method is a strategy that ‘increases the odds’ and ‘allows for significant profits’, making it an accessible and practical money system for any player.
Disadvantages of the Monte Carlo Method
In the previous explanations, we highlighted that the Monte Carlo method is a useful strategy for players seeking to increase their win rate or focus on profit.
Of course, no strategy is perfect in all respects (as all strategies do not affect the expected value), and the Monte Carlo method also has its disadvantages. The following section outlines the disadvantages of the Monte Carlo method and suggests ways to mitigate them.
Because it involves a sequence of numbers, it is cumbersome to use and prone to mistakes
The primary disadvantage of the Monte Carlo method, particularly for beginners, is that it can seem tedious.
Indeed, the Monte Carlo method requires managing a sequence of numbers to adjust bets up or down. Compared to simpler strategies, such as the Martingale or Parlay methods, which merely double the bet, the Monte Carlo method involves significantly more steps and is more prone to errors.
For this reason, it is recommended to practise using free play until you become familiar with the method, or to use table games like roulette that have no betting time limit. Additionally, always use written notes to track the number sequence rather than relying solely on mental calculations.
Repeated winning and losing increases the amount of bets
| Sequence | Bets | Win/Loss |
|---|---|---|
| 1・2・3・4・5・6・7・8・9・10 | $11 | Win |
| 3・4・5・6・7・8 | $11 | Loss |
| 3・4・5・6・7・8・11 | $14 | Win |
| 5・6・7 | $12 | Loss |
| 5・6・7・12 | $17 | – |
If you win and lose repeatedly without reducing the number sequence sufficiently, the betting amount can rise sharply after losses. This is evident when examining examples.
Suppose, for instance, that you win after a sequence of ‘1 2 3 4 5 6 7 8 9 10’ in a 3x payout game. In this case, the next sequence becomes ‘3 4 5 6 7 8’, requiring a $11 bet. If you lose, the sequence grows to ‘3 4 5 6 7 8 11’. Following another win, the sequence reduces to ‘5 6 7’, but another loss changes it to ‘5 6 7 12’.
Thus, while the leftmost number disappears upon winning, repeated wins and losses without reducing the sequence length can cause bet amounts to climb rapidly.
Prone to long-term battles
The Monte Carlo method, though defensive, has a tendency to lead to prolonged games. In contrast, similar defensive strategies, such as the Martingale and Cocomo methods, can conclude a set with a single win. With the Monte Carlo method, consecutive losses extend the number sequence, prolonging the game.
For example, in a triple payout scenario, you may need to win up to three times, while in a double payout scenario, you must win at least twice unless your initial bet succeeds. This results in slower profit accumulation.
The Monte Carlo method is best suited for players seeking steady, consistent profits. If your goal is to make quick gains, alternative strategies may be more suitable.
Measures are needed for double dividends
As mentioned briefly earlier, using double dividends can sometimes result in no profit, even if a number sequence is completed with fewer than one number remaining.
To ensure profit, one approach is to divide the remaining numbers into smaller parts and continue with the method. For instance, if the number ‘5’ remains, you could split it into ‘2’ and ‘3’ and proceed.
However, continuing with the sequence as it stands is also acceptable, as the losses are unlikely to become significantly negative.
How to Use the Monte Carlo Method to Increase Your Win Rate
Finally, this section explains how to use the Monte Carlo method more effectively to increase your win rate. The Monte Carlo method can be employed in a variety of games, including roulette (triple payouts) and baccarat or sic bo (double payouts).
As a strategy that balances advantages and disadvantages, it is widely used. However, using the Monte Carlo method strategically can help you achieve better results.
Cutting losses and forcing a reset during losing streaks
The Monte Carlo method leads to an ever-increasing number sequence during losing streaks. If consecutive losses persist, the sequence may grow to 20 or 30 numbers, resulting in mounting losses and prolonged recovery periods.
To avoid unsustainable losses, it is wise to force a reset if the sequence becomes too large. Establish a predetermined threshold for resetting, such as 12–15 units for 3x payouts or 10 units or fewer for 2x payouts.
Combining with the empty roulette wheel
Many players use the Monte Carlo method for roulette, as it accommodates both triple and double payouts. Roulette outcomes are independent events, so there is no predictive basis for assumptions like “it was red 10 times in a row, so it will be red again” or “it hasn’t been in the 1–12 dozen for 15 spins, so it’s overdue.”
Instead, approaching the game calmly and analysing trends can lead to better decisions. For example, in non-live roulette, you might make an empty turn, or in live roulette, observe several rounds before placing bets. This measured approach reduces errors and increases your chances of success.
Monte Carlo Method: Legends and Trivia
Finally, here is some trivia about the Monte Carlo method.
The Monte Carlo method was originally developed in land-based casinos (overseas storefront casinos) and is a money system surrounded by many legends. In this article, one of the legends associated with the Monte Carlo method is explained.
Is the Monte Carlo System derived from the name of a collapsed casino?
‘Monte Carlo’ refers to a district in the Principality of Monaco, a small European country, where casinos are a major attraction.
According to one theory, a group of players who employed this strategy made so much money playing roulette and other games that they bankrupted the casino. This story gave rise to the legend known as the Monte Carlo strategy.
While the Monte Carlo method is considered a useful strategy among casino enthusiasts, this legend lacks credibility. It is unlikely that a casino would go out of business solely because a group of players used this strategy.
Summary
The Monte Carlo method is a relatively defensive strategy that focuses on increasing win rates and can be profitable in various situations, provided there are no consecutive losing streaks.
With a 3x payout, it succeeds in winning more than once in five attempts, making it an efficient and profitable betting system. However, its disadvantages include the complexity of adjusting betting amounts and the extended time required to recover from a losing streak.
It is a strategy that can lead to a higher winning rate if bets are placed calmly and with a clear understanding of when to quit.









